When an object is placed on an inclined plane, various forces come into play that affect its motion.
The primary force causing the object to slide down is gravity, which pulls it toward the Earth. This gravitational force acts to accelerate the object down the slope, especially as the incline becomes steeper.
As the block slides down the inclined plane, it experiences both gravitational force and friction.
The degree of tilt of the surface determines how quickly the object will slide. A steeper incline results in a greater component of gravitational force acting parallel to the slope, thus increasing the object’s speed.
Understanding the interaction of these forces is key to grasping the motion of objects on inclines.
Readers will discover more about how different angles and surfaces influence movement and what happens when the forces are balanced or unbalanced. This exploration unveils the fundamental principles of physics that govern everyday experiences, from skiing to car racing.
Fundamentals of Inclined Plane Dynamics
Inclined planes are essential in understanding how objects move on tilted surfaces.
Key concepts include the forces acting upon an object, the role of gravity, and the decomposition of weight components into parallel and perpendicular forces.
Understanding Inclined Planes
An inclined plane is a flat surface that is tilted at an angle to the horizontal. This setup allows objects to slide down due to gravity.
The angle of the incline affects how easily an object moves. A steeper angle leads to a greater gravitational force acting parallel to the surface, resulting in faster acceleration. This principle is important in many real-world applications, from ramps used in loading docks to the design of roller coasters.
The Role of Forces on an Incline
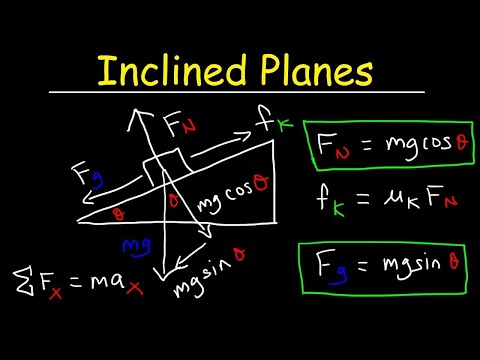
Two main forces act upon an object on an incline: the force of gravity and the normal force. Gravity pulls the object downward, while the normal force acts perpendicular to the surface.
When analyzing motion on an incline, it’s essential to decompose these forces into their components.
The gravitational force can be broken down into a parallel component that drives the object down the slope and a perpendicular component that counteracts the normal force. The interplay between these forces determines whether an object will slide down the incline, remain stationary, or accelerate.
Decomposing the Weight Component
To understand motion on an incline, it is crucial to decompose the weight into its components.
The force of gravity acts directly downward, but its effects change on a slope. Trigonometry is often used here.
The parallel component (Fg, parallel) can be calculated using Fg, parallel = mg sin(θ), where m is mass, g is the acceleration due to gravity, and θ is the angle of the incline.
The perpendicular component (Fg, perpendicular) acts against the normal force and can be expressed as Fg, perpendicular = mg cos(θ).
Analyzing these components through a free-body diagram clarifies how weight affects the motion and helps predict the behavior of the object on the inclined plane.
Physics of Motion Down an Incline

The motion of an object sliding down an incline is influenced by several key factors, including the forces acting on the object, the angle of the incline, and the effects of friction. Understanding these concepts helps explain how gravity and friction determine the speed and behavior of objects on inclined surfaces.
Friction and Motion
Friction plays a major role in the motion of an object on an incline.
There are two main types of friction to consider: static and kinetic friction.
Static friction prevents the object from moving initially, while kinetic friction acts when the object is already sliding.
The force of friction can be calculated using the formula:
Friction Force (F_f) = Coefficient of Friction (μ) × Normal Force (N)
The normal force is the perpendicular force acting on the object. On an incline, the normal force is less than the object’s weight, which affects the friction force.
A higher coefficient of friction leads to a greater friction force, slowing the object down as it slides down the incline.
Acceleration and Velocity
When an object slides down an incline, it experiences acceleration due to gravity.
The gravitational force can be broken into two components: one parallel to the incline and one perpendicular to it.
The net force on the object can cause it to accelerate:
Net Force (F_net) = Gravitational Force (F_g) – Friction Force (F_f)
If the gravitational force exceeds the friction force, the object accelerates down the incline.
The object’s velocity depends on the angle of the incline and the forces acting on it. The steeper the angle, the greater the component of gravitational force acting downward, leading to increased acceleration.
Effects of Various Angles and Surfaces
The angle of the incline significantly impacts motion. As the angle increases, the component of gravitational force acting parallel to the incline also increases.
This results in a higher acceleration and faster sliding down the incline.
Different surfaces have different coefficients of friction. For example, a smooth, frictionless incline allows the object to slide without resistance, resulting in maximum acceleration.
On the other hand, a rough surface with a high coefficient of friction will generate a larger friction force, slowing the object down.
Understanding these factors helps predict how an object will behave on varying incline angles and surface types.
